Incompressible flows, interface capturing and cavitation
Staff:
Incompressible flow
Standard time-marching compressible flow solvers used extensively in the aerospace industry cannot be applied incompressible flows, as the governing equations lack a time derivative in the continuity equation. For steady flows the artificial compressibility method modifies the incompressible flow equations to allow standard time marching to be used. The dual-time technique further extends the artificial compressibility approach to allow time accurate calculation of unsteady incompressible flows.
At Bristol we have produced 2D and 3D time accurate incompressible versions of industry standard flow solvers on both fixed and moving meshes. Images below show streamlines for incompressible flow in a lid driven cavity.
Re=100-240x200.png)
Re=400-240x200.png)
Re=1000-240x200.png)
(a) Re=100 (b) Re=400 (c) Re=1000
The new single phase incompressible codes developed at Bristol, were used as a foundation upon which to build a multiphase solvers. Further research was carried out into free-surface flow and cavitating flows.
Interface capturing including free surface flow
 Numerical simulations of flows with a moving interface separating two immiscible fluids are complex. The numerical simulation of two fluid flows, particularly water and air flows are central to many different types of applications, including the flow through rivers and aqueducts, and is crucial in the design and construction of naval hydrodynamics, examples of which include ships, submerged bodies, oil platforms and buoys. Various numerical techniques have been developed to predict the motion of an interface between two fluids. The main approaches that have been developed can be characterised as surface-fitting, surface-tracking and surface-capturing. Surface fitting methods involve adapting the topology of the computational mesh to match the interface position. The use of surface fitting maintains a high interface resolution, however such methods are limited to surfaces that do not undergo large deformations. Large deformations in the interface would lead to significant distortion of the computational mesh, which degrade solution convergence and quality.
Numerical simulations of flows with a moving interface separating two immiscible fluids are complex. The numerical simulation of two fluid flows, particularly water and air flows are central to many different types of applications, including the flow through rivers and aqueducts, and is crucial in the design and construction of naval hydrodynamics, examples of which include ships, submerged bodies, oil platforms and buoys. Various numerical techniques have been developed to predict the motion of an interface between two fluids. The main approaches that have been developed can be characterised as surface-fitting, surface-tracking and surface-capturing. Surface fitting methods involve adapting the topology of the computational mesh to match the interface position. The use of surface fitting maintains a high interface resolution, however such methods are limited to surfaces that do not undergo large deformations. Large deformations in the interface would lead to significant distortion of the computational mesh, which degrade solution convergence and quality.
 Previous results have shown that even simple interface geometries can produce large mesh deformations. Another disadvantage of surface fitting methods is the need to recompute the metrics of the computational mesh after each mesh adaption, which can be computationally costly depending upon the implementation used. Surface-tracking and surface-capturing schemes both use meshes that do not move to conform to the free surface; hence the grid related issues of surface-fitting schemes are avoided. Surface-tracking methods explicitly track the interface location through marker points or functions. The reconstruction of the free surface location which is required for such methods can be complex, especially in three dimensions. The surface-capturing approach treats the interface as a contact discontinuity in the density field that is captured by the numerical scheme along with the velocity and pressure fields. This approach is analogous to shock capturing in single phase compressible flow. The need for complex surface tracking and reconstruction procedures is eliminated and complicated interface motion can be predicted. The use of such schemes on an arbitrarily unstructured mesh is no more complicated than on its structured counterpart. Furthermore, this approach can readily handle situations of fluid break-up, entrapment, reconnection and also situations in which the interface develops as part of the flow solution provided the computational mesh resolution is sufficiently fine for the case in question.
Previous results have shown that even simple interface geometries can produce large mesh deformations. Another disadvantage of surface fitting methods is the need to recompute the metrics of the computational mesh after each mesh adaption, which can be computationally costly depending upon the implementation used. Surface-tracking and surface-capturing schemes both use meshes that do not move to conform to the free surface; hence the grid related issues of surface-fitting schemes are avoided. Surface-tracking methods explicitly track the interface location through marker points or functions. The reconstruction of the free surface location which is required for such methods can be complex, especially in three dimensions. The surface-capturing approach treats the interface as a contact discontinuity in the density field that is captured by the numerical scheme along with the velocity and pressure fields. This approach is analogous to shock capturing in single phase compressible flow. The need for complex surface tracking and reconstruction procedures is eliminated and complicated interface motion can be predicted. The use of such schemes on an arbitrarily unstructured mesh is no more complicated than on its structured counterpart. Furthermore, this approach can readily handle situations of fluid break-up, entrapment, reconnection and also situations in which the interface develops as part of the flow solution provided the computational mesh resolution is sufficiently fine for the case in question.
At Bristol, a central difference formulation with added dissipation has been developed for interface capturing. This represents a direct extension of the widely used Jameson scheme developed for compressible flow and previously extended at Bristol to single phase incompressible flows via an artificial compressibility approach. Care is needed to ensure that the cell-centred scheme correctly balances the source term and the hydrostatic pressure flux.
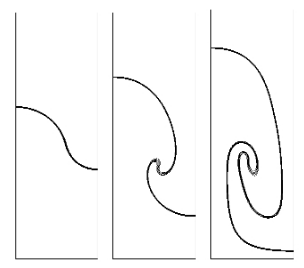
Free surface profile for submerged hydrofoil (Left) Contours for the Rayleigh-Taylor instability (Right)

Cavitation
Cavitating flows occur in many situations in fluid dynamics. Examples are high speed submerged bodies, propellers, nozzles and hydrofoils. Cavitation is similar to boiling, but differs due to the thermodynamic paths taken by the liquid which precede vapour formation. Cavitation will occur in a liquid when the local pressure falls sufficiently below the vapour pressure of the liquid, in contrast to the boiling of a liquid which will occur when the vapour pressure rises above the ambient pressure due to a thermodynamic input. Cavitation generally produces unwanted side effects such as noise and damage. However, cavitation can be used beneficially, for example cavitation on hydrofoils reduces the overall drag. Cavitation also finds applications in other scientific areas including ultrasound cleaning and sonochemistry.
The complexity of cavitating flows presents considerable modelling difficulties for computational methods. Cavitation introduces physical changes to the initial liquid which mean that two-phase flows with high density ratios; discrete interfaces; and non-equilibrium interfacial mass and momentum interactions arise. Dissolved gases and turbulence are important in the cavitation process and must be considered appropriately for the flow under consideration. Further important issues include: unsteadiness; compressibility; the state of the boundary layer and surface roughness, which can change the phase change threshold.
Sheet cavitation is the term used to describe a single vapour filled attached region of cavitating flow that forms on a solid surface and appears stable in a quasi-steady sense For such flows a number of simplifications to a full two phase model, required for most cavitating flows, can be made: these simplifications significantly reduce the high computational costs. In the methods implemented at Bristol, a incompressible isothermal homogeneous mixture model with interface capturing has been used, with a diffusion or transport equation for the concentration of the gaseous phase. This is suitable for cavitation in water at room temperature. The time-averaged incompressible Navier-Stokes equations for the mixture are coupled with Spalart-Allmaras and k-omega turbulence models. A central difference finite volume time-accurate artificial compressibility scheme, with preconditioning, is used, which is based on the standard Jameson scheme.
Cp for hemisphere/cylinder, Spalart-Allmaras turbulence model, Cavitation number=0.3


t=0.24,(b)t=0.26-400x96.png)
t=0.28,(d)t=0.30-400x96.png)
t=0.32,(f)t=0.34-400x96.png)
t=0.36,(h)t=0.38-400x96.png)